Iterates of multidimensional approximation operators via Perov theorem

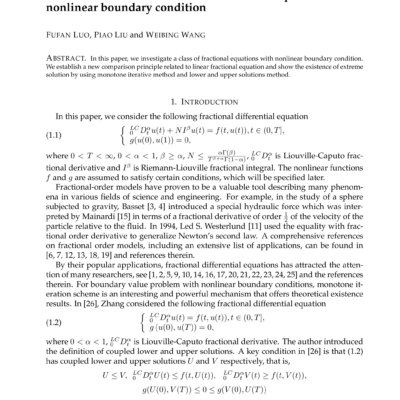
The starting point is an approximation process consisting of linear and positive operators. The purpose of this note is to establish the limit of the iterates of some multidimensional approximation
operators. The main tool is a Perov’s result which represents a generalization of Banach fixed point theorem. In order to support the theoretical aspects, we present three applications targeting
respectively the operators Bernstein, Cheney-Sharma and those of binomial type. The last class involves an incursion into umbral calculus.