Multi-step inertial proximal contraction algorithms for monotone variational inclusion problems

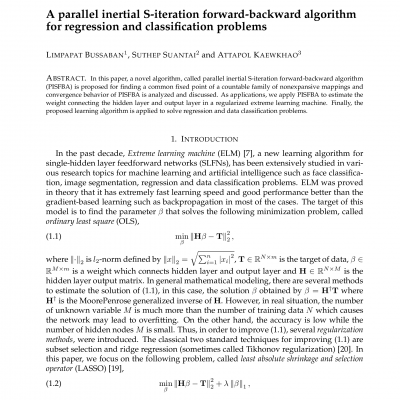
In this article, we introduce the multi-step inertial proximal contraction algorithms (MiPCA) to approximate a zero of the sum of two monotone operators, with one of the two operators being monotone and Lipschitz continuous.
The weak convergence of the MiPCA is shown under the summability condition formulated in terms of the iterative sequence in a Hilbert space setting. We also investigate the unconditional convergence of the one-step inertial proximal contraction algorithm. Finally, numerical experiments are given to illustrate the advantage of the multi-step inertial proximal contraction algorithms.