» Ideals in residuated lattices

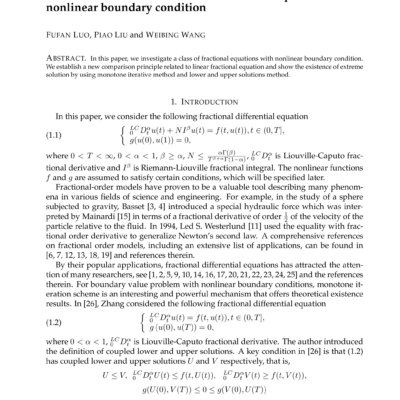
The notion of ideal in residuated lattices is introduced in [Kengne, P. C., Koguep, B. B., Akume, D. and Lele, C., $\mathcal L$ -fuzzy ideals of residuated lattices, Discuss. Math. Gen. Algebra Appl., 39 (2019), No. 2, 181–201] and [Liu, Y., Qin, Y., Qin, X. and Xu, Y., Ideals and fuzzy ideals in residuated lattices, Int. J. Math. Learn \& Cyber., 8 (2017), 239–253] as a natural generalization of that of ideal in $MV$ algebras (see [Cignoli, R., D’Ottaviano, I. M. L. and Mundici, D., Algebraic Foundations of many-valued Reasoning, Trends in Logic-Studia Logica Library 7, Dordrecht: Kluwer Academic Publishers, 2000] and [Chang, C. C., Algebraic analysis of many-valued logic, Trans. Amer. Math. Soc., 88 (1958), 467–490]). If $A$ is an MV algebra and $I$ is an ideal on $A$ then the binary relation $x\sim _{I}y$ iff $x^{\ast }\odot y,x\odot y^{\ast }\in I$ , for $x,y\in A,$ is a congruence relation on $A.$ In this paper we find classes of residuated lattices for which the relation $\sim_{I}$ (defined for MV algebras) is a congruence relation and we give new characterizations for i-ideals and prime i-ideals in residuated lattices. As a generalization of the case of BL algebras (see [Lele, C. and Nganou, J. B., MV-algebras derived from ideals in BL-algebras, Fuzzy Sets and Systems, 218 (2013), 103–113]), we investigate the relationship between i-ideals and deductive systems in residuated lattices.