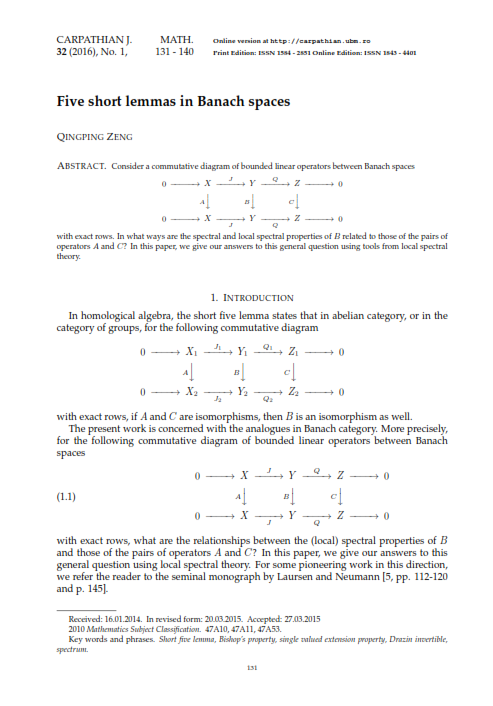
carpathian_2016_32_1_131_140_abstractcarpathian_2016_32_1_131_140Consider a commutative diagram of bounded linear operators between Banach spaces
![\[ \begin{CD} \qquad\qquad\qquad\qquad 0 @>>> X @>{J}>> Y @>{Q}>> Z @>>> 0\\ @. @V{A}VV @V{B}VV @V{C}VV @. \qquad\qquad\qquad \qquad \\ \qquad\qquad\qquad\qquad 0 @>>> X @>>{J}> Y @>>{Q}> Z @>>> 0 \end{CD} \]](https://www.carpathian.cunbm.utcluj.ro/wp-content/ql-cache/quicklatex.com-5a2932a0c3a948f7e28f6df894e24433_l3.png "Rendered by QuickLaTeX.com")
with exact rows.
In what ways are the spectral and local spectral properties of 
related to those of the pairs of operators 
and 
?
In this paper, we give our answers to this general question using tools from local spectral theory.
| Author(s) | Zeng, Qingping |
|---|
An inertial method for solving the split equality fixed point problem with multiple output sets
Asymptotic modeling of non-linear viscopiezoelectric Kelvin-Voigt type plates via Trotter theory
Properties of isocompact spaces in topological groups
Two generalized cyclic projection algorithms for solving a class of the split feasibility problem in real Hilbert spaces
Global-fixed-point property of gyrogroup actions
On simple normal structure and best proximity points in reflexive Banach space
Unified Convergence Analysis of Certain At Least Fifth Order Methods
A set packing model for the Partition Coloring Problem
Cut-through connections of graphs
Positive solutions for multipoint boundary value problem of fractional differential equation with parameter
